Nan Hua Primary School PSLE Math Prelim 2006 (Q48)

X and Y are two schools in the same cluster. In school X, the ratio of the number of girls to the number of boys is 4 : 1. In school Y, the ratio of the number of girls to the number of boys is 2 : 3. School X has twice as many pupils as school Y.

a) Find the ratio of the number of girls in school X to the number of boys in school Y.

b) After 40 boys leave school X to join school Y, the ratio of the number of girls to the number of boys in school Y becomes 5 : 8. How many boys are there in school Y now?


Solution

Q(a)



Answer: The ratio of the number of girls in School X to the number of boys in School Y is 8 : 3.


Q(b)

40 boys from School X joined School Y



The number of units for boys increased by 1 unit from 15 to 16.

1 unit ----- 40 (boys transferred to School Y)

Total number of boys in School Y

16 units ----- 16 x 40 = 640


Answer: There are 640 boys in School Y now.

Teachers' pay to be pegged closer to performance

Teachers' pay to be pegged closer to performance
Dec 28, 2007

OUTSTANDING teachers and principals will get better pay and bonuses from next year, when their salaries will be pegged more closely to their performance.

From April, annual increments will no longer be fixed at three per cent. Instead, the 29,000 officers in the education service can expect up to a five per cent increment depending on their performance and potential.

Bonuses based on merit will also vary according to whether teachers are graded 'good', 'very good' or 'outstanding'.

'Good' performers will receive a bonus of up to two months' extra pay, while those who are 'very good' will get up to 3.25 months.

'Outstanding' performers can take home up to 4.25 months' more.


There are pluses and minuses in any performance related pay. Appraising a teacher’s performance is subjective. My opinion is that the MOE should make clear as to what constitutes good, very good, outstanding, average and below par performance.

My guess is that MOE is going to allow schools to have the liberty to set its own standards and criteria. There lies the problem. Effectively, this would be a system handed down by the HQ, only for the details to be ironed out by individuals at ground level. This would only cause non-uniform standards to be applied to teachers in different schools who will be rewarded by an employer, with a supposed uniform standard.

Any comments on performance related pay for school teachers?

Related Articles (updated on 7 Jan 2008):
Performance-linked pay more harm than good
Another Article on Teachers' Pay

Nan Hua Primary School PSLE Math Prelim 2006 (Q47)

At first, Jonathan had 2/3 as many stamps as Kevan. After Jonathan bought another 8 stamps and Kevan lost 5 stamps, Jonathan now has 4/5 as many stamps as Kevan. Find the number of stamps Jonathan had at first.


Solution



(Jonathan) 2 units + 8 ----- 4 parts

2 units ----- 4 parts – 8



1 unit is half of 4 parts - 8

1 unit ----- 2 parts – 4 *


(Kevan) 3 units – 5 ----- 5 parts

3 x (2 parts – 4) – 5 ----- 5 parts *

6 parts – 12 – 5 ----- 5 parts

6 parts – 5 parts ----- 12 + 5

1 part ---- 17

Note(*) – 1 unit --- 2 parts – 4,
therefore, 3 units --- 3 x (2 parts – 4)


Jonathan at first

4 parts – 8

= (4 x 17) – 8

= 68 – 8

= 60

Answer: Jonathan had 60 stamps at first.

Nan Hua Primary School PSLE Math Prelim 2006 (Q45)

In the figure below, O is the centre of the circle. ABCD is a square and OD is 14 cm. Quadrant OAC is equal to Quadrant BAC. Find the area of the shaded parts.

Solution
Reconfiguring the figure ….


Angle EOC ----- Angle FOC + Angle FOE

= 90 degrees + 45 degrees

= 135 degrees

Area of EOC is therefore ----

135 degrees divided by 360 degrees --- 3/8 of whole circle


Shaded area of circle is
Area of whole circle – Area of EOC – Area of Triangle AOC


(22/7)(14)(14) square cm – (3/8)(22/7)(14)(14) square cm – (1/2)(14)(14) square cm

= (616 – 231 – 98) square cm

= 287 square cm

Answer: The shaded area is 287 square cm.

Nan Hua Primary School PSLE Math Prelim 2006 (Q44)

A box containing 3 files weighed 10.2 kg. Peter added 2 more files and 3 books into the box and the mass of the box with its contents became 19 kg. If the mass of one file was four times the mass of the book,

a) Find the mass of the box.
b) Peter could only lift a maximum mass of 13 kg. What was the least number of files that he could remove from the box so that he was able to lift the box?


Solution

Mass of 1 file was four times mass of the book.

File ----- 4 units
Book ----- 1 unit


Box with 3 files weighed 10.2 kg

Box + (3 x 4 units) ----- 10.2 kg

Box + 12 units ----- 10.2 kg



Peter added 2 files and 3 books, mass now 19 kg.

Box + 5 files + 3 books ----- 19 kg

Box + (5 x 4 units) + 3 units ----- 19 kg

Box + 20 units + 3 units ----- 19 kg

Box + 23 units ----- 19 kg


In summary,

Box + 12 units ----- 10.2 kg
Box + 23 units ----- 19 kg

Box with 23 units is heavier than Box with 12 units by, 19 kg – 10.2 kg = 8.8kg

This 8.8 kg is caused by the extra 11 units in Box with 23 units, as compared to Box with 12 units.

Therefore,

11 units ----- 8.8 kg

1 unit ----- 8.8 kg divided by 11 = 0.8 kg


Q(a) Mass of box is

Box + 12 units ----- 10.2 kg

Box + (12 x 0.8kg) ----- 10.2 kg

Box + 9.6 kg ----- 10.2 kg

Box ----- 10.2 kg – 9.6 kg = 0.6 kg

Answer: The box weighed 0.6 kg.


Q(b) Least number of files

1 file ---- 4 units x 0.8 kg = 3.2 kg.

If he removed 1 file, the total mass would be

19 kg – 3.2 kg = 15.8 kg


If he removed 2 files, the total mass would be

19 kg – 3.2 kg – 3.2 kg = 11.6 kg.

11.6 kg is less than 13 kg, but 15.8 kg is more than 13 kg.


Answer: Peter needed to remove 2 files so that he was able to lift the box.

Nan Hua Primary School PSLE Math Prelim 2006 (Q39)

School P has 180 more pupils than School Q. If 60 pupils are transferred from School Q to School P, there will be 4 times as many pupils in School P as School Q. How many pupils are there in School P?

Solution




3 units ----- 60 + 180 + 60 = 300

1 unit ----- 300 divided by 3 = 100

School P ----- 4 units – 60
= (4 x 100) – 60
= 340

Answer: There are 340 pupils in School P.

Three-quarters of Singapore teachers hold degrees

An article from the Straits Times dated 25 Dec 2007, inferring that graduate teachers will produce better students.

Three-quarters of Singapore teachers hold degrees

Do you agree? There appears to be mixed opinions, judging by the comments that follow the report.

An Article on GEP

Found an interesting article in the Forum Section of the Straits Times (24 December 2007).

Gifted scheme: Has it achieved the set goals?

Excerpts below:

THE Gifted Education Programme (GEP) was introduced by the Ministry of Education (MOE) in 1984. It sees the participation of the top 1 per cent of each Primary 4 cohort (about 500 pupils) every year.

Special teaching and learning techniques have been used to groom these top pupils in the hope of achieving the following goals, as stated by MOE on its website……….

……It has been 23 years now since the implementation of the GEP. If the intake had been constant at 500 per year, there should be 11,500 of such GEP graduates and students to date.
And those in the first batch would be 33 years old now.

It is believed among experts that by the age of 30, top-performing individuals would have flourished in their careers.

Could the MOE comment on how well it has achieved the goals set by itself with regard to GEP students? They are remarkable students with extraordinary school grades.

Tremendous efforts are put in by the GEP teachers and educationists to groom these bright kids. Taxpayers' money has been pumped in to craft the best syllabus to develop our creme de la creme.

Can MOE highlight the significant contributions made to society by the working GEP cohort……….

Click on the link above for the full article.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q44)

Michael and Luke had 600 stickers altogether. After Michael gave away 40 of his stickers, 2/5 of the number of Luke’s stickers was equal to 2/3 of Michael’s stickers.

a) How many stickers did Michael have at first?

b) How many stickers did Luke buy so that the ratio of the number of Luke’s stickers to the number of Michael’s stickers is now 7 : 3?

Solution



8 units + 40 ----- 600

8 units ----- 600 – 40 = 560

1 unit ----- 560 divided by 8 = 70


Q(a)

Michael had at first

3 units + 40 ----- (3 units x 70) + 40

= 210 + 40 = 250

Answer: Michael had 250 stickers at first.


Q(b)

After Michael gave away 40 stickers and before Luke bought some stickers, the ratio of the number of Luke’s stamps to the number of Michael’s stamps was 5 : 3.

After Luke bought some stamps, the ratio was 7 : 3.



Luke bought 2 units ----- 2 units x 70 = 140

Answer: Luke bought 140 stamps.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q47)

In a bookshop, 64% of the books are Chinese books. The rest are Malay and Tamil books. The ratio of the number of Malay books to the number of Tamil books is 3 : 1. There are 110 more Chinese books than Tamil books.

a) What percentage of the books are Malay books?
b) How many Chinese books are there?


Solution



4 units ----- 36%

1 unit ----- 36% divided by 4 = 9%

(Chinese books) – (Tamil books) ----- 110 books

64% - 9% ---- 110 books

55% ----- 110 books

1% ----- 110 books divided by 55 = 2 books


Q(a)

(Malay books) 3 units ----- 3 units x 9% = 27%

Answer: 27% of the books are Malay books.


Q(b)

(Chinese books) 64% ----- 64 x 2 books = 128 books

Answer: There are 128 Chinese books.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q48)

At a birthday party, Mr Chew had a bag of sweets. If each child receives 5 sweets, he is left with 6 sweets. If each child receives 7 sweets, he would be short of 4 sweets.

a) How many children are there at the party?

b) How many sweets did Mr Chew give to each child if he is left with only 1 sweet?


Solution




Q(a)

For 1 child --- if Mr Chew gives 5 sweets instead of 7, he ends up with 2 “more” sweets.

2 “more” sweets ----- 1 child

1 “more” sweet ----- 1 divided by 2 = ½

10 “more” sweets ----- ½ x 10 = 5

Answer: There were 5 children at the party.



Q(b)

If Mr Chew gave the 5 children 5 sweets, he would have 6 sweets left.

Number of sweets he has is therefore

(5 children x 5 sweets) + 6 sweets left = 31 sweets


If he is left with just 1 sweet, it means he gave away 30 sweets to the 5 children.

30 sweets divided by 5 children = 6 sweets each

Answer: He gave 6 sweets each.

Tuition or No Tuition? (Part 3) – Tutoring your own child

There are a few things that need to be considered if you decide to tutor your own child. Besides allocating your precious time (lots of it) for your child, you need to consider the fact that you have to study her schoolwork too. The last thing you’d like to happen is to teach her something which you were taught 30 years ago, only to find that what you were taught then, is no longer applicable in schools today!

Here are the points you need to note if you decide to tutor your own child:


1. Time Allocation for your child – A Very Important Factor

You need to have a fixed time allocated for your child if you decide to tutor her. Your time (lots of it), must be set aside on a daily basis. There is NO two ways about it. If you can’t afford that time for her, you will be shortchanging her.

You cannot expect to tutor her just an hour or two a week, and expect results. To her, you are a parent, not a tutor. As such, her expectation from you, as her parent, is far more demanding than that from a paid tutor. She sees you as THE role model. She sees you as a moral guide.

A paid tutor is expected to be with your child only once or twice a week. However, as a parent, if you tutor her only once or twice a week, she will perceive that you are only interested in her work, once or twice a week. As such, if you decide to tutor her, you need to do it everyday.

How much time you are able allocate depends whether you are working or not. A full time housewife is most ideal. She would be in command to dictate the time schedule. However, not all parents are full time homemakers. If that is the case, you need to manage your time between work and time for your child.

Whatever the case, you will need anywhere between 2 hours to as much as 4 hours per day with your child. Sounds demanding? No. That’s parenting.


2. Be in the know what the school syllabus is

This sounds logical. But unfortunately, many parents do not bother to find out what is taught in schools. By now, many parents know that algebra is no longer used to solve Maths problems in Primary schools. However, because many parents do not keep in touch, they are unable to solve Maths problems at P5 and P6 today.

You also have to keep abreast of changes in the syllabus. For example, only this year (2007), for the topic of the Solar System (P5 Science), Pluto is no longer considered one of the planets. The decision taken by MOE was made after textbooks were published for the Year 2007. Parents who were not aware of this, kept teaching that the Solar System has 9 planets, when it was revised that it has only 8!

For the next academic year 2008, the use of calculators is introduced at P5. P6 will continue with the old system where no calculators are allowed at all. This means that assessment books that were published for P5 Maths before this year, are all tailored for the old system, ie without the use of calculators. The parent who wishes to tutor his or her own child, must be aware of this.


3. Master the subject content – another “must do” action

Nothing is more frustrating to a student than a teacher or tutor who does not know the content he or she is teaching! Likewise, if a parent decides to tutor his or her own child, and if he or she does not know the content, it demoralizes the child.

In order to be able to teach, you must know the content. Equip yourself with the latest answering techniques in Maths problems. If you want to guide English, your grammar must be strong, your vocabulary must be wide and your ability to form properly constructed, grammatically correct sentences, must not be in question. You must also be able to explain under what conditions certain tenses can be used, and under what conditions those tenses cannot be used.

If you wish to guide your child in Science, not only you must know the content, you must also know the details of the whys. You must know key words, key concepts and why certain answers will get students full marks, while other answers score only partial marks.


4. Test your own subject content competency and ability to teach

A good way to test your own competency is to “sit” for the Exam Papers yourself, within the stipulated time. If you find that there are many questions you are not able to answer yourself, or if you fail to complete the paper within the stipulated time, you know that you are not competent enough to sit for the paper, let alone teach your child.

That is half the test. The other half of the test is the teaching itself. Assume you did well for the Exam Papers. Now pass your work to your child and see if she understands your answers and working. If she does, good. If she does not, are you able to explain to her such that she understands what, how and why you answered the way you answered?

If she still is unable to understand the what, how and why you answered the way you answered, then the problem is not your competency in the subject per se, but perhaps, your teaching methods. You may be teaching a method that you were taught 30 years ago, which is no longer applicable in primary schools today – eg Algebra.


5. Make sure you teach what is taught in schools

You have to teach in the same manner as the school actually teaches – not what you think how it should be taught. For example, if the school expects models and statements for Maths, you have to teach that. If you try to bypass that and use your own “creative method”, your child may lose marks in exams and that may demoralize her.

Some schools expect intermediate statements in their Maths workout. These statements explain the steps taken when a calculation is done. You have to teach your child that too, if the school insists students write intermediate statements.

Other schools insist that every step of the working be shown. Short cuts are not allowed and the student gets penalized if she jumps steps. If that is the case, you have to make sure your child does not jump steps.


6. Oral Test – The Most Neglected Section in the English Exam

The Oral section is the most neglected section in the English Paper. Most schools hardly stress this section, while most tuition centres and tutors ignore this section completely.

Oral is a small part of the English Paper. However, it can be very unnerving for the student who does not get enough practice for this section. Unlike written exams, oral exams are taken in actual real-time, “face-to-face” exams.

A mistake made in a written exam can be corrected later. A mistake during an oral exam cannot be retracted. Once a mistake is made, the student’s confidence dives and she will be very nervous for the rest of the Oral Test. This may have a psychological impact on the student and further pressure her in her written exam that is to come.

It is therefore very important that enough oral practice is given, not just to get the reading aloud right, but the psychological mindset of the student in “prepared mode”.

If you tutor your own child, you must know what the examiner looks for during the oral test. To equip yourself, it would be advisable to get from retail bookshops, books that give you tips on the oral section of the English Exam.


7. Science – easy to learn, but teaching it is a different story

If you were to read P3 to P6 science textbooks and try out the assessment papers yourself, within a matter of weeks, you would have covered the whole PSLE Science syllabus. There is not much content in PSLE Science, as compared to the other subjects.

Teaching the subject to your child is a different matter altogether. You have to know what are the key words and concepts. Some assessment books in the market come with Answer Keys that highlight key words and concepts. Others do not. You have to choose your resources carefully. Failing to highlight the important keywords and concepts to your child will cost her a lot of marks in her exams.


8. The Child’s Discipline – a “must have”

This means that you have to allocate a specific time for her tuition with you. No TV, no computer, no music playing in the background, no phone chats, no distraction during your tuition time with her. On a regular basis, you also have to give assessment papers, which must be done within the stipulated time.

It must be remembered that during an exam, your child will have no luxuries like music, friends to chat or tidbits to bite. If your child does not have the discipline to complete the 2 and one-quarter hour Maths paper under such a condition at home, what makes you think she will have that required discipline in school during a real exam?

It is therefore important for your child to get accustomed to exam conditions well before the real exam. Without discipline, every thing falls apart. You, as your child’s tutor, will have to see that her discipline is in place.


9. Your Discipline – an even bigger “must have”

If Dad decides to teach his son, but halfway through the lesson, he proceeds to watch the live match on ESPN, do you think son will be motivated to work?

If Mummy decides to teach her daughter, but halfway through the lesson, she calls her friend over the phone to have a chit-chat, do you think daughter will be motivated to work?

Children are humans too. When the boss does not care, don’t expect the subordinates to be motivated to do their best. Likewise, if parents do not show full commitment, the child won’t be too committed either.

That said, it is OK if dad or mum does “real work”. For example, if dad assigns the child to complete a two and one-quarter hour Maths Assessment Paper, and dad completes his own project work on his computer beside his son, that is acceptable. Likewise, mum may decide to complete her ironing chores in the same room, while the child does her work quietly.

Children are intelligent and reasonable. They know if dad and mum do “productive work” or are just excusing themselves for their own leisure.


10. The biggest plus – your ability to reward, punish and create bonding

As a parent, if you are your child’s own tutor, the biggest plus is that you are able to decide – and no one is able to override you – what kind of reward or punishment your child gets. The hands of a teacher or an external tutor are tied. Yours are not.

As a parent, you know your child’s likes and dislikes. You can reward her if she does her work dutifully with what she likes. Likewise, you can withhold what she likes if you feel her work is unsatisfactory.

As a parent, if you do all the above correctly, your child will respect you – for life. The biggest spin-off about tutoring your own child is the parent-child bond you create – and that relationship would most likely be carried into adolescence and even into their young adulthood.


Conclusion

Nobody said parenting is easy. It is one of the heaviest responsibilities in life. Whether you decide to tutor your own child depends on a whole host of factors.

The final decision is for the parents to make.

This is the end of a 3-part series of "Tuition or No Tuition".

=====================

Related articles
Tuition or No Tuition? (Part 1)
Tuition or No Tuition? (Part 2)

The Present Tenses

There are 2 types of Present Tenses, the Simple Present Tense and the Continuous Present Tense.

1. Simple Present Tense –

This tense is used when we talk about permanent situations or events that happen regularly or all the time.

Examples –

a) Nurul lives in Toa Payoh.

b) Water freezes at 0 degree Celsius.

c) The sun sets in the West.


2. Continuous Present Tense -

This tense is used when we talk about a temporary or continuing action that is “currently happening”.

Examples –

a) Mother is cooking dinner.

b) Boon Keng is studying in his room.


3. Identifying the difference between Simple Present and Continuous Present Tenses –

a) Maniam works hard.

b) Maniam is working hard.

In the first example, the reader gets the impression that Maniam is a hardworking person. The Simple Present Tense, “works”, infers that Maniam works hard on a regularly basis.

In the second example, the reader gets the impression that Maniam is currently working hard. Whether he is working hard now because he has always been a hardworking person, or he is working hard now only and this is just a one-off action, the reader will not be able to tell.


4. Grammar Rules pertaining to the Simple Present Tense (Subject-Verb Agreement)

The singular noun goes with the singular verb while the plural noun goes with the plural verb.

Example of the singular noun, singular verb - Charlene dances gracefully.

Example of the plural noun, plural verb – The girls dance gracefully.


Identifying the singular noun -

Some students have difficulty in identifying the singular noun.

Collective nouns are considered singular nouns – eg a bouquet of flowers, a class of pupils etc.

- A class of pupils is going to the Botanical Gardens. (correct)

- A class of pupils are going to the Botanical Gardens (wrong)


Uncountable Nouns are considered singular nouns – eg water, sand, ice, air etc are considered singular nouns, and hence, will be followed with the singular verb.

- Air is important to all living things.

- Glass is fragile.


Abstract Nouns are considered singular nouns – eg anger, joy, envy.

- Anger is bad for your health.

- Knowledge is important.


In summary, there are 2 types of Present Tenses. The Simple Present Tense is used when we talk about a regular and/or permanent event, while the Continuous Present Tense is used when an event is “currently” happening. At the same time, we must be aware of the subject-verb agreement, where if the noun is singular, the verb that follows it has to be in the singular form.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q43)

During a travel fair, 120 families chose Switzerland as their holiday destination. 80% of the remaining families chose Australia while the rest chose Japan. If 10% of all the families at the travel fair chose Japan, how many families were there at the travel fair?


Solution




20% of remaining families ----- 10% of all families

10% of remaining families ----- 10% divided 2 = 5% of all families

(Australia) 80% of remaining families ----- 8 x 5% = 40 of all families


(Australia) 40% of all families + (Japan) 10% of all families ----- 50% of all families

Therefore Switzerland is also ----- 50% of all families, which makes up 120 families.


50% ----- 120 families

100% ----- 120 families x 2 = 240 families

Answer: There were 240 families at the travel fair.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q42)

Tap X can fill a tank in 4 minutes. Tap Y can fill a similar tank in 6 minutes.

a) What fraction of the tank is filled when Tap X is turned on after 1½ minutes?

b) What fraction of the tank is filled after one minute when both taps are turned on at the same time?

c) How long will it take to fill up this tank when both taps are turned on at the same time?


Solution

Q(a)

Full Tank (Tap X) ----- 4 min

In 1½ min, it can fill up ----- 1½ min divided by 4 min = 3/8

Answer: It can fill up 3/8 of the tank in 1½ min.


Q(b)

(Tap X) 4 min ----- 1 tank

1 min ----- 1 divided by 4 = ¼ tank

(Tap Y) 6 min ----- 1 tank

1 min ----- 1 divided by 6 = 1/6 tank

Both taps on at the same time

1/4 + 1/6 = 5/12

Answer: 5/12 of the tank will be filled if both taps are turned on at the same time after 1 min.


Q(c)

Tap X takes 4 min to fill up whole tank.
Tap Y takes 6 min to fill up whole tank.

If both taps are turned on at the same time, the ratio of the volume that would be filled by Tap X to the volume filled up by Tap Y would be



(Tap X) Full tank ----- 4 min

3/5 filled ----- 3/5 x 4 min = 2.4 min

(The other 2/5 is filled by Tap Y, hence after 2.4 min, the tank would be full)

Answer: It takes 2.4 min or 2 min 24 sec for the tank to be full if both taps are turned on at the same time.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q40)

Lamp posts are placed round a square field measuring 60 m by 60 m. The lamp posts are placed 10 m apart with a post at each corner. How many posts are needed in all?

Solution




1 side of square has

7 lamp posts – 2 corner lamp posts = 5 lamp posts.

4 sides ----- 5 lamp posts x 4 = 20 lamp posts

Total lamp posts ----- 20 lamp posts + 4 corner lampposts = 24 lamp posts

Answer: There are 24 lamp posts in all.

Tuition or No Tuition? (Part 2)

This is a continuation of last week’s article Tuition or No Tuition? (Part 1)

This week, we will take a look at what are the points parents have to look for, when they sign up their child for tuition. Here are some issues parents need to note.


1. My child has had many tutors. Yet, there still is no improvement in his performance.

Some parents think tutors are magicians. Just because the tutor has the qualifications, does not mean that your child can produce the results you want. Could it be that your child is disinterested in the first place?

Parents must take note that any relationship between two people takes time. The same goes for tutor-pupil relationship. If you keep changing tutors every few weeks or months, you may not give the tutor and pupil enough time to build a close and cordial relationship.


2. The tuition centre my child attends has been producing many A* students. Why then is my child not producing A* for his subjects?

Firstly, parents must remember that each child’s ability is different. Some are good at studies. Others are good at sports, while still others may be strong in the humanities area like art, music, dance etc. No two children are the same.

Secondly, it could be that many of the students who attend this centre are bright students to start with. If that is the case, and if your child is average, in all probability, the tutor has been tailoring his lessons to suit his bright students – which means your child is lagging behind.

Rather than enrolling your child in an “A* producing tuition centre”, your child would be better off being tutored in a centre, where the ability of his peers is around his ability.


3. Almost every other centre claims to have marked improvements in their students’ scores. Are they believable?

Rest assured, they tell you the truth. But hold on there, there is more to it. It is a “secret” within the education industry, that students who are performing well will stay with the centre, while students who don’t perform, will leave. This is what is called the “natural attrition of weak students”.

Thus, by the end of the academic year, the bright students who stayed with the centre, will produce the results the centre can be proud of. At the same time, the non-performing students would have left the centre months before the year end. This then causes the centre to have a high percentage of students with high scores and improved results - which the centre proudly tells you.

So next time you see an advertisement that claims that such and such a centre produces high scoring students with improved marks, just take note the subtle silence on their attrition rate.


4. My child is weak in Science only, but the centre (or tutor) does not teach Science only.

Because it is easier to teach Maths than any other subject, you can find many tutors and centres that teach Maths. English tutors are a little more difficult to find than Maths tutors, because teaching English requires a different set of skills. While the skills to teach Maths can be acquired in a relatively short time, the skills needed to teach English are not so easily acquired. As for Science, qualified science tutors are the most scarce.

The law of economics in the education industry applies, just as with any other service or industry. If you need a subject where few tutors specialize in that area, you need to pay a premium price.

However, if you do not wish to match the price for a “Science only” tutor, you will have to expect Maths being thrown in with Science. The demand for “Maths only” among students is highest, while the demand for “Science only” is lowest.

There is a high economy of scale teaching Maths only, but a low economy of scale teaching Science only. It therefore is not very economical for a centre, or a tutor, to teach Science only.

As mentioned, it is all a matter of economics. If you are willing to pay the price to have a “Science only” tuition, the tutor will be willing to do the job.


5. My child is weak only in certain topics in a certain subject. Where can I find a tutor to tailor his lessons to my child’s needs?

Again, economics apply. The demand for specific topics within a subject is much less than the demand for any other combination of subject needs. This means for the tuition centre or tutor, it is not economical to concentrate on specific topics only. However, my guess is that there are tutors out there who will be willing to tailor their lesson plan to your child’s needs – with a premium price, that is.

One thing parents must realize is that if the child is weak only in certain topics, it may be more feasible to get him to ask the teacher to explain to him the topic again. It is the teacher’s job to teach. So it is the right of the student to ask a teacher for clarification or further explanation. Tuition in this case may not even be needed.


6. But I insist my child to have tuition so that he can maintain his A* in his subjects!

Well, as a parent, you know his capabilities. If you feel your child has that capability and he enjoys all the tuition you give him, all the best to him in his endevour to attain a high PSLE score.


This concludes Part 2 in the series “Tuition or No Tuition?”. Next week, in Part 3, we will discuss issues you have to be aware of, if you, the parent, decide to tutor your own child.

====================================

Related article:
Tuition or No Tuition? (Part 1)
Tuition or No Tuition? (Part 3) – Tutoring your own child

Slow Download

To all visitors,

Apologies for the slow download time the last 24 hours or so, from the morning of 12 Dec to the next morning on 13 Dec.

Looks like the glitch is fixed for now. Let's hope it stays that way.

Regards,
Excel Eduservice

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q39)

Two identical big squares A and B overlap to form a small square of area 36 square cm. The ratio of the shaded area to the unshaded area is 1 : 6. Find the length of x.

Solution


(Shaded Area) 1 unit ----- 36 square cm.
(Unshaded Area) 6 units ------ 36 square cm x 6 = 216 square cm.


Area of 1 large square is
(216 square cm divided by 2) + 36 square cm
= 144 square cm.

Length of side of large square is

144 square cm = 12 cm x 12 cm


Answer: The length of x is 12 cm.

Anglo-Chinese School (Primary) P5 SA2 2006 Math Question (Q37)

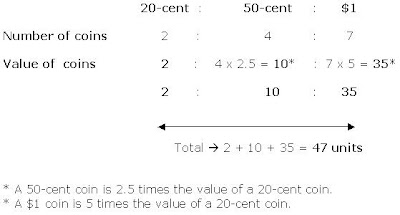
The ratio of the number of 20-cent coins to the number of 50-cent coins to the number of $1 coins in a bag is 2 : 4 : 7. Given that the total amount of money in the bag is $47, how many coins are there altogether?

Solution



47 units ----- $47 (Total amount of money in bag is $47)

1 unit ----- $47 divided by 47 = $1

Value of 20-cent coins ----- 2 units ----- $2
Value of 50-cent coins ----- 10 units ----- $10
Value of $1 coins ----- 35 units ----- $35

No. of 20-cent coins ----- $2 divided by 20 cents ----- 10 coins
No. of 50-cent coins ----- $10 divided by 50 cents ----- 20 coins
No. of $1 coins ----- $35 divided by $1 ----- 35 coins


Total number of coins in bag

10 + 20 + 35 = 65

Answer: There were 65 coins in the bag.

Has the Mystery of Stonehenge been solved?

Watch this incredible video of how one man moves tons of blocks and created a mini Stonehenge in his backyard. The simplest of tools and knowledge of science were used - levers and gravity.




The topic of Simple Machines is taught in Primary 5 Science. It is a PSLE Science Topic.

One of the machines in the P5 Science syllabus is the lever. One of the functions of the lever is to help us move heavy objects. When the fulcrum is placed nearer to the load, compared to the effort, only a small effort is needed to move a larger load.



The video is a testimony that a small force can move heavy objects with the help of the simple lever.

Note that in the "see-saw" scene, which uses the lever principle, a small load is added on one side of the beam so that one side of the beam tilts towards the ground. The builder then raises the fulcrum and the beam is hence, raised.

In the scene where the beam is raised vertically, again, a small load is placed on one side of the beam, causing it to tilt towards the vertical position. Again, the principle of the lever is used.


In an earlier early scene where the builder moves the block in a circle, the principle of the lever is used yet again. He places a rock (the fulcrum) near the centre of the block (the load). In this case, the load is in between the fulcrum and the effort, unlike the other two examples, where the fulcrum is in the centre.

Again, note that the distance between the load and fulcrum is still much smaller than the distance between the effort and the fulcrum, enabling a small effort to move a much larger load.

An illustration is shown below.




Some information about Stonehenge.

http://dictionary.reference.com/browse/stonehenge

Stone·henge
a prehistoric monument on Salisbury Plain, Wiltshire, England, consisting of a large circle of megaliths surrounding a smaller circle and four massive trilithons; dating to late Neolithic and early Bronze Age times (c1700–1200 b.c.) and believed to have been connected with a sun cult or used for astronomical observation

How do I handle the Class Brat?

He’s every teacher’s nightmare. He disrupts the class, he distracts other students, he’s Mummy’s boy – and he’s in YOUR class. Touch him once, and Mummy’s coming to get you thrice. So, what do you do?

It all depends on what the scenario is. Let’s face it. It is wonderful to have the 'ideal class' where all 40 students pay attention every single minute. But we all know that even in the best of classes, the brat exists. So rather than lament that the brat is in YOUR class and not in another teacher’s class, some pro-active action needs to be taken.

If you have a supportive principal and/or vice-principal, it makes life so much easier for you. The problem is that this is not the case all the time. When this happens, the best thing is for you to contact the parents.

Here are points to consider:

1. Calling Parents of the disruptive student gives you the upper hand -

When you have an 'untouchable' brat in class, you can expect his parents to be over-protective over him. The slightest 'punishment' from you, example making him stand in the corner of the class, may result in you being 'summoned' for 'wrongful' punishment. It is therefore advisable on the teacher’s part to call the parents about his behaviour, before they call you – or worse, your principal.

If you initiate the call, it puts you on the platform that you are the teacher and you are in charge – and their child has violated some basic class rules. If they call you, it puts you on the defensive, and you now have to explain and convince them, why you have 'punished' their child.

If you make the call, you place yourself as 'the authority'. You, as a teacher, have made it known that teaching is your job and if their child disrupts the class, he is disrupting you (a civil servant and a government official) from performing your duties delegated to you, by the government.

If they call you, they expect you to accede that you treat their (brat) child in a special manner, and if you don’t, they will get you (a civil servant and a government official) 'reported' to the authorities.

In short, the party who makes the call, calls the shots.


2. Teaching is your job, parenting is the parents’ job –

Some parents expect their child to be treated as the special one in class. They say that their child is special. But then, every child is special, isn’t it? What they mean is that they expect YOU, to parent their child in their absence. A subtle hint to them that you are the teacher - (which means your job is to teach, and they are the parents, which means that their job is to nurture the child) - will usually drive home the point that they cannot expect “special favours”.

Some parents may insist that their child have the “right” to behave in such a manner in class because he is “different”. What can be done is to “reverse” the situation. Would they be happy if their child is eager to learn in class, only to be distracted by his classmates?

Some students not only disrupt, they also shout at teachers and use vulgarities at his classmates and schoolmates. You can put forward a simple question to his parents like, “Mr and Mrs ….., do you think that cursing or using swear words is a socially acceptable behaviour?”

It would take a nutty parent to answer “yes” to the above question. More often than not, when that question is posed, the parents will remain silent out of embarrassment, because you have actually hinted that they, as parents, have failed to raise their child properly.


3. Build rapport with the parents, and the job’s more than half done –

It is so much easier to control the disruptive student if you have his parents’ support. It is therefore important that you build a rapport with his parents and convince them that they need to be involved.

It must be remembered that it is the parents who have the ultimate ability to motivate, reward and punish, their own child. As such, if you convince the parents that their child is not behaving in a socially acceptable manner, you can expect them to take action that their child puts effort to be less disruptive in class.

So what if the parents are not supportive? That happens too, doesn’t it?

The “worst case scenario” is when you have a brat in class, your principal is not as supportive as you wish he would be, and the parents don’t support the idea that his unacceptable behaviour should be curtailed. Now what?

The above situation hardly happens. But if it does happen to you, just remember that the child will only stay with you at most, 2 years.

Every job has its ups and downs. Be thankful that the class you have keeps changing every year or every two years. If you work in the private sector, that brat kid may be in the form of the brat colleague who is the boss’ pet – and he may well stay with you for much longer than 2 years!

Teaching is such a wonderful job. It is stressful, yes. But come on now, which job isn’t?

Tao Nan School P5 SA2 2006 Math Question (Q48)

At an exhibition, 30% of those present were boys. 5/14 of the remainder were girls and the rest were adults. There were 45 more adults than boys. How many more children than adults were there at the exhibition?

Boys ----- 30%

Girls ----- 5/14 of remainder

= 5/14 x 70% = 25%

Total children ----- 30% (boys) + 25% (girls) = 55%


Adults ----- 100% - 55% (children) = 45%

There were 45 more adults than boys, therefore,

45% (adults) – 30% (boys) ----- 45 people

15% ----- 45 people

1% ----- (45 people) divided by 15 = 3 people


Children more than adults -----

55% (total children) – 45% (adults) = 10%

10% ----- (3 people) x 10 = 30 people


Answer: There were 30 more adults than children.

Tuition or No Tuition? (Part 1)

It is the end of the academic year and the annual process starts again. Tuition shopping time! That’s the big headache for today’s parents. It seems that every parent would like their child to have a headstart. So they go round “shopping” for the "best" tuition centre or tutor. Having taught students both as a schoolteacher and a tutor, I can perhaps give parents a tip or so, on whether your child needs tuition or not.


The School Teacher – the best judge for your child

Parents, your child's teacher is your child’s best friend. She knows where are your child’s potential, weak points, strong points and areas that need to be improved. She sees your child, 5 days a week, 40 weeks a year.

Besides you (and the domestic maid, if you employ one), your child’s teacher is probably the only other adult your child sees almost everyday without fail. That itself, makes your child’s teacher an important person in your child’s life.

I am not propagating that you should call the teacher up every single day. She, like you, has a family too. However, there are many things she knows about your child which you may not even know! A good time to get to know the teacher is during the “Meet the Parents” session. But you don’t have to wait for that. There is nothing to stop you from taking a day off to meet the teacher if such a need arises.

The teacher will be able to advise you if your child is weak in a certain area. She will be able to give you feedback if your child has been doing his homework or not. It must be remembered that the child is YOUR child. To the teacher, your child is just another student out of the 40 she has in her class. So the onus is on you to make effort to know your child’s teacher, rather than wait for her to call you - because if she does call, more often than not, it would be news you’d rather not hear.


The Teacher’s advice is most likely to be objective with no bias –

You pay school fees to the government. The government pays the teacher her salary. This makes her an excellent person to consult on matters about your child. She does not worry if she tells you the truth that your child does not need tuition, or if he needs it. Her pay does not depend on whether you take up tuition or not. Hence, you can be assured that she has no vested financial interest, if she were to advise you that your child needs tuition.

The advice given will be based on her opinion as a teacher. She will be able to tell you if your child is weak or capable. Furthermore, she will be able to tell you which specific area, or topic, your child needs to pay attention to. With this advice, you will be in a better position to make a decision if your child needs tuition or not.

If your child is weak in only one subject, it means there is no need to give him tuition for all subjects. If you give him tuition in areas he does not need, you are burdening your child with extra work.

If your child is weak in a certain topic within a subject, it may be advisable to engage a one-to-one tutor to strengthen that particular topic, and that topic only. Again, there is no necessity to burden your child with extra work.

If your child is weak in all subjects, check out if the whole class is also weak in all subjects. If that is the case, in all probability, there will be extra lessons for the whole class – which means, free tuition from the expert, which is the teacher herself.

So before you burden your child with more tuition, find out if he needs it in the first place. If you have not been talking to his teacher, make it a point that you do that the next academic year. However, please bear in mind that this does not mean you can call her 24/7. After all, the teacher, like you, needs to have her own private life too.


This is Part 1 of the article “Tuition or No Tuition?”. In Part 2, we will discuss the points that you need to be aware of, should you decide to give your child tuition.

That would be posted next weekend.

============================

Updated:
Tuition or No Tuition? (Part 2)
Tuition or No Tuition? (Part 3) – Tutoring your own child

S'pore education system works - with tuition (ST Forum)

Straits Times Forum 30 Nov 2007

S'pore education system works - with tuition

THE Minister of State for Defence, Associate Professor Koo Tsai Kee, praised the Singapore education system in his article, 'Singapore's education system works' (ST, Nov 24). There are two issues which need clarification.

Prof Koo commented that 'without the GEP, many outstanding students from working-class families in neighbourhood schools would not have been able to move to the good schools'. Is he implying that neighbourhood schools which do not offer the Gifted Education Programme (GEP) are not good schools? That all GEP schools are good schools?

The annual Primary 1 registration is already stressful for both parents and schools. Some parents, eager to put their children in 'good' schools, clocked up numerous hours in volunteer work, only to find that they did not have the luck of the draw. Then there are parents whose children had already secured places in such schools. Fearing that their little ones might not be able to cope with the demands of the school, or to give their kids a leg up, they enrolled them in enrichment programmes and, thence on, it is tuition all the way till the PSLE.

Implying that neighbourhood schools are inferior to GEP schools is being very unfair to non-GEP schools and their teachers. Moreover, this puts additional pressure on some parents to get their children into GEP schools.

Prof Koo concluded his article by pronouncing that the Singapore education system is one that works. I do not refute this, but there is a missing addendum - with tuition.

It is a well-known fact that a large proportion of our students, from pre-primary all the way to pre-university, have tuition.

Some children are so reliant on tuition that even though they score above-average grades, they would insist on continuing with tuition the following year, for 'security' reasons.

Students in schools with the integrated programme (IP) are supposedly the cream of the crop, yet there are many who have tuition. A few centres have been called 'IP tuition centres' because of their large number of IP students.

When a child says that he does not have tuition, but his parents coach him using assessment books, that is tuition in a sense.

So, Singapore's education system does work - with tuition.

Ng Kim Yong (Mrs)


Do you agree with Mrs Ng that Singapore's education system is highly dependent on tuition?

Feel free to give your comments.

Maha Bodhi School SA1 2004 Math Question

A teacher has a bag of sweets. If she gives each pupil in her class 5 sweets, she will have 20 sweets left. If she gives them 8 sweets each, she will need to buy another 88 sweets. How many pupils are there in the class?

Solution



For every pupil that is given 8 sweets instead of 5, the teacher needs 3 more sweets.

From the above model, it can be seen that if she gives 8 sweets to each pupil instead of 5, she would need 108 more sweets.

3 “more sweets” ----- 1 (pupil)

1 “more sweet” ----- 1/3

108 “more sweets” ----- 1/3 x 108 = 36

Answer: There are 36 pupils in the class.

Thinking Skills in Science

In this post, the topic of Magnets will be discussed to demonstrate how thinking skills is used.

Knowledge -
A magnet has two poles, a north-pole (N) and a south-pole (S). Like poles repel, while unlike poles attract.

Example:

Using the above knowledge to think and deduce –

Question:
Three metal bars A, B and C, are placed on a table. Only two of the metal bars are magnets, while the third is not. Hazri experimented the 3 metal bars as illustrated below.
Which of the two metal bars are magnets?



Answer: Metal Bars A and C are magnets. Metal Bar B is not.

Thinking Process –

When like poles of 2 magnets face each other, the magnets will repel. In Experiment 3, Metal Bars A and C repel. From this, we can conclude that the like poles of Metal Bars A and C were facing each other and hence, the bars repelled.

In Experiments 1 and 2, although the metal bars were attracted to each other, it does not mean that the bars are magnets. You need only one bar to be a magnet, and the other bar to be a non-magnet made from magnetic material (such as iron, steel or nickel), for the bars to be attracted to each other.

Can you solve this? Are you able to identify the pole that is marked “X” on Magnet E?

Answer: South-pole. Do you know why?

Here is the reason.

Tao Nan School P5 SA2 2006 Math Question (Q47)

Ricci saved $200 from her salary and spent the rest. She spent 1/9 of the expenditure on a blouse, $40 on a scarf and the rest on books. The amount spent on the scarf was $20 less than that spent on the blouse. What was her salary?

Solution




She spent $20 less on the scarf than on the blouse.

Scarf cost $40, therefore blouse cost $40 + $20 = $60


(Blouse) is 1/9 of expenditure ----- $60

(Total expenditure) 9/9 ----- $60 x 9 = $540

Salary ----- $540 (total expenditure) + $200 saved = $740

Answer: Her salary was $740.

Tao Nan School P5 SA2 2006 Math Question (Q46)

Cherie had 3 times as much money as Jolene. After Cherie spent $40.00 and Jolene spent $8.00, Jolene had 3 times as much money as Cherie. How much money did Cherie have at first?

Solution





Cherie before spending

9 units + $8 +$8 +$8

= 9 units + $24


Cherie after spending ---- 1 unit

Cherie spent $40, therefore,

Amount she had before spending – Amount she had after spending ----- $40

(9 units + $24) – 1 unit ----- $40

8 units + $24 ----- $40

8 units ----- $40 - $24 = $16

1 unit ----- $16 divided by 8 = $2


Amount of money Cherie had at first

9 units + $24

= (9 x $2) + $24

= $18 + $24

= $42

Answer: Cherie had $42 at first.

How do I motivate the class? Part 2

Last week we took a look at what are the most common reasons why some students perform badly in PSLE Maths. How do I motivate the class? Part 1

This week, we will be discussing how to improve the student’s performance in English.

English, compared to Maths, takes a much longer time before significant improvement in marks can be seen. While a 20 mark increase is possible within 3 months for students who are weak in Maths, any significant improvement in English can only be observed anywhere from 6 months to a year.

With Maths, what is needed is a basic foundation and practice, practice, practice. With English, it is a different ball game altogether. It is no coincidence that students who read a lot, tend to do much better in English than students who hardly read.

Regular reading helps the students recognize sentence patterns and structures – the key to grammar. At the same time, with wide reading, the students are exposed to a diverse group of words, expanding their vocabulary.

It can be argued that a student, who has weak grammar skills and a weak vocabulary database, will do poorly for his English paper. Many English teachers advise students that they have to read a wide range of books, journals etc.

Weak grammar and vocabulary will lead to poor performances in areas like Continuous Writing and the Comprehension Sections. It is therefore important to strengthen the student’s grammar and vocabulary.


Weak Link Number 1 - Grammar

Some students do not know the rules of basic grammar like subject-verb agreement. If this is not corrected early, there will be more problems later. If the student cannot write grammatically correct sentences involving the Simple Present Tense, eg “He walks to school” and not “He walk to school”, he will have problems writing grammatically correct sentences when more complex tenses like Perfect Tenses are introduced.

Unlike the past, students today are not taught grammar rules in a structured manner. However, there is nothing to stop the English teacher from teaching structured Grammar Lessons in his or her class. From experience, if the class has a very weak foundation in grammar, that seems to be the only plausible thing to do.

Teaching Grammar Lessons however, can be boring, as compared to teaching themes. The challenge is to keep the class attentive when you drive home the grammar rules. However, that can be done if you add a little humour to your lessons.

For example, if you were to introduce the Present Perfect Tense, you can get the students involved. In the following example, let us assume that we are teaching the Present Perfect Tense. Let us get a student, Charles, involved.

After explaining the form of the Present Perfect Tense (have/has + past participle) and the conditions that have to be met for the tense to be used, we can draw a cartoon image to liven the lesson.


Click on image below to enlarge


In the drawing above, the structure and the use of the Present Perfect Tense has been emphasized. In this case, the condition for the use of the Present Perfect Tense, that is an action or event that has recently happened, is drilled to the students.

With this manner of teaching, the boredom of teaching grammar is eliminated.


Weak Link Number 2 – Vocabulary

Some students add “new words” to their vocabulary list. That is good. The problem is that they end up with tons of “new words”, but do not know how to use them!

Hence, for every “new word” the student learns, it is good for him to copy the whole sentence from where he found that “new word”.

For example, noting that the word “practice” is a noun, while “practise’ is a verb, is basic vocabulary. However, this understanding can be further enhanced, if the student gets to see sentences constructed with those words in it. Example -

“The sports practice will begin at 3 pm. We will practise the 100-metre sprint.”

In the above, the use of the words “practice” and “practise” are both used in sentences. This gives the student a better idea how those two words differ in meaning, compared to just an explanation that “practice” is a noun, while “practise” is a verb.


The Other Sections in the English Paper

Grammar and Vocabulary form a major part of the English Paper. If the student does not get these two basics right, he will do badly for the subject. Having noted that, certain sections are notoriously problematic for the average student. One section where most students lose marks is the Open Ended Comprehension. Another section is Cloze Comprehension.

Just like Maths where multiplication tables, fractions and decimals are the basics - in English, Grammar and Vocabulary are the basics. If the student does not get his basics right (ie grammar and vocabulary), we can expect his other sections to be affected too.

For Maths, with close coaching, we can see an improvement in the student’s performance within as short as 2 or 3 months. For English, the process is longer. It may take up to a year, before we get to see any significant improvement in marks.

Whatever the case, be it Maths, English or any subject, if the student consistently fails in his subject(s), it can be a source of demotivation.

In order for the student to be motivated, the best way is for him to produce the results he can be proud of.

This is the end of a 2-part article “How do I motivate the class?”

Next week, we will feature another article – “How do I handle the class brat?”

=====================

Related link: How do I motivate the class? Part 1

Ai Tong School P6 CA1 2004 Math Question

Tap A took 3 min to fill up a tank. Tap B took 4 min to fill up the same tank. However, if you pulled out the plug at the bottom of the tank, the tank could be emptied in 12 min. If both the taps are turned on and the plug pulled out at the same time, how long would it take for the tank to be filled up?


Solution

Tap A takes 3 min to fill up tank, while Tap B takes 4 min.

That means that if both taps are turned on at the same time, the ratio of the volume of water in the tank that will be filled by Tap A to the volume of the water in the tank filled by Tap B would be 4:3, because Tap A has a higher rate of water flow.

Volume of Water in Tank filled



Total ----- 7 units

For the tank to be filled by both taps,
Tap A will fill up ----- 4 units divided by 7 units = 4/7 of the tank.

But we know that Tap A takes 3 min to fill up whole tank.

Hence, for Tap A to fill up 4/7 of tank ----- 4/7 x 3 min = 12/7 min.

Therefore, the tank will be full in 12/7 min when both taps are on because 4/7 of the tnak will filled up by Tap A, and the other 3/7 by Tap B.


Rate of water flowing in

12/7 min ----- 1 tank

1/7 min ----- 1/12 tank

1 min ----- 7 x 1/12 tank = 7/12 tank


Rate of water flowing out

12 min ----- 1 tank

1 min ----- 1/12 tank


When both taps are turned on with plug pulled out

Water flowing in – Water flowing out

1 min ---- 7/12 tank – 1/12 tank = 6/12 or 1/2 tank.

Since 1/2 tank takes 1 min to be filled, 1 tank will take 2 min.

Answer: It takes 2 min for tank to be fully filled if both taps are turned on, and with plug pulled out.

Many still cannot speak or write English properly

Many still cannot speak or write English properly

Excerpt from the Straits Times Forum, dated 3 Dec 2007.

I WAS pleased to read the report, 'Singapore pupils shine in global reading test' (ST, Nov29). If I understand correctly, the test was of silent reading and comprehension. I also note that English-language experts warned schools here not to be complacent and that our students lag behind many others in spoken and written English.

Click on the link above for the full report.

Anglo Chinese School 2004 PSLE Math Prelim Question

The ratio of the number of marbles received by John and Peter was 4:7 respectively. The ratio of the number of marbles received by Peter and Sam was 9:5. John gave 1/12 of his marbles to Sam. Peter gave 1/9 of his marble to Sam. Eventually, Sam had 135 marbles.

(a)Find the ratio of the number of John’s marbles to Sam’s marbles at first.
(b)Find the total number of marbles received by the 3 boys.


Solution

Q(a)


Answer: The ratio of the number of marbles to Sam’s is 36:35



Q(b)

John ----- 36 units
Peter ----- 63 units
Sam ----- 35 units

John gave 1/12 to Sam
Peter gave 1/9 to Sam
Eventually, Sam had 135 marbles

John ----- 1/12 x 36 units = 3 units to Sam
Peter ----- 1/9 x 63 units = 7 units to Sam

Sam eventually had ----- 35 units + 3 units + 7 units = 45 units

45 units ----- 135 marbles
1 unit ----- 135/45 = 3 marbles


Total number of marbles received by 3 boys

(John) 36 units + (Peter) 63 units + (Sam) 35 units
= 134 units total

134 units ----- 134 x 3 marbles = 402 marbles altogether.

Answer: The boys received 402 marbles altogether.